Power and energy of the Tohoku tsunami: Revised estimates.

Last March 12, a day after the Tohoku earthquake and tsunami, I posted an estimate of the power and energy in the tsunami, based on a rough estimate from observations at the time. My conclusion was that the energy was about 30 kilotons, roughly equivalent to the energy in the combined bombs that were dropped on Hiroshima and Nagasaki (36 kt). I felt that the energy could have been a factor of ten or a hundred higher given uncertainties in the height of the waves and duration of the event. Thanks to a reader's inquiry, here are some updated numbers based on data that has since emerged.

(Please give credit to this blog site if you use the numbers posted below!)

First, let me summarize the method.  Professor Michael McIntyre of the University of Cambridge calculated the power in a tsunami using Bernoulli's theorem, and concluded that under certain conditions the power in a tsunami is about 1 megawatt per meter of shoreline, or 1 gigawatt per kilometer.  The conditions are that the tsunami has a height of 1 meter in the open ocean, a velocity of 220 meters per second. I used these data directly and assumed a similar power, then estimated the length of shoreline attacked (one-half of the east coast of Honshu) and the duration of the tsunami (100-1000 seconds).  The model applies to tsunamis on the open ocean.

There are still not a lot of data available, but the velocity of 220 meters a second for the speed of the tsunami is in the right ballpark to account for the time between the earthquake and the time that it took for the tsunami to reach the coast (30-60 minutes).  The major uncertainty here is the height of the tsunami on the open ocean. I used the value of 1 meter that is typically used for tsunamis (because they are typically not even noticed on the open ocean). There were two ocean-bottom sensors in place that measured a height of 7 meters (Maeda, Takuto et al., Earth Planets Space, 63, 2011, in press) where the water was 1618 m and 1013 m deep respectively, and so I'll use a new value of 7 meters for the open-ocean height of the tsunami.

McIntyre's calculation of the power in the tsunami was based on an ocean depth of about 4.5 kilometers--deep open ocean.  In order to make my calculation internally consistent, I need to use shallower depth of, say 1.5 kilometers.The power, as calculated by McIntyre, is proportional to the depth to the three-halves power.

The height of the tsunami comes into the calculation as h-squared; the depth of the ocean as a square root.  Thus, the smaller ocean depth would reduce the power by a factor of 1.7 (sqrt 4.5/2) and the greater height of the tsunami would increase it by a factor of 49 (7 squared). The combined effects cause an increase of 28.8 in the power (let's round it up to 30).

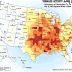
To get energy from this, I assumed that the event lasted 100-1000 seconds, and that the length of coastline affected was about 1300 km. Both assumptions still seem reasonable. If you look at the map above of wave heights impacting Honshu, the northern half of the island, which is the half that I originally assumed was affected, has significantly greater wave heights than the southern half. Furthermore, for the model to be internally consistent, the number I really need is the length of the tsunami out on the open ocean not the amount of shoreline impacted. However, the assumption that the length of the tsunami was about equal to the length of the northern half of the island should give a ballpark estimate of the length at sea.

To summarize: I would increase my original estimate of the minimum power from  1.3*10^12 watts or 1.3 petawatts to 40 petawatts, and my original estimate of minimum energy to 40*10^14 joules or 930 kiloton, which could easily be rounded up to 1 megaton. These are the power and energy for an event of 100 seconds duration.  I believe that a more realistic duration is 1000 seconds, giving 400 petawatts and 10 megatons as the preferred values.  Note: Please See the first comment by a reader. Suggests that something between the min and max would be a better number to use.  So, perhaps best to say "a few hundred petawatts" and "a few megatons" given all of the uncertainties. 

 (For comparison, the 400 Pw and 10 Mt values are about 280 times the the combined energy of the bombs that destroyed Hiroshima and Nagasaki (15+21 kilotons.) The energy of the lateral blast at Mount St. Helens was about 24 megatons.)

Please see reader comments!